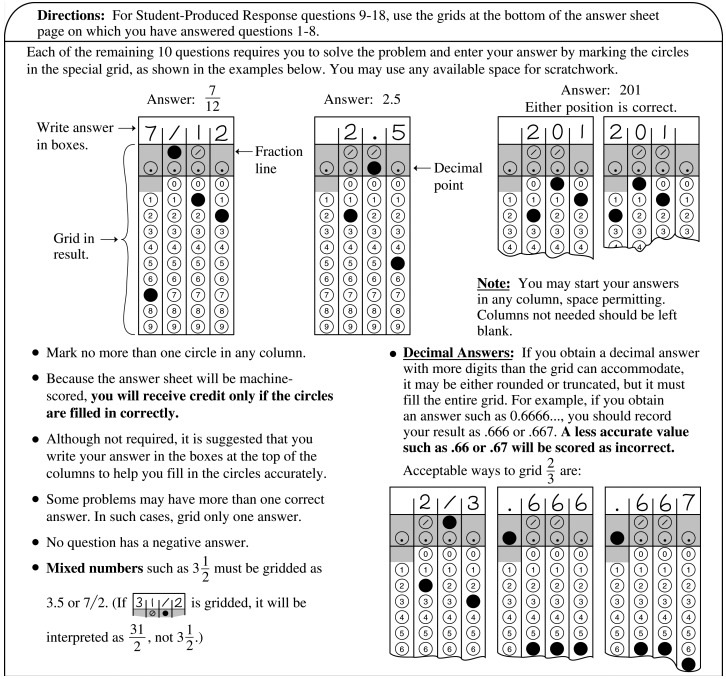
Questions of this type have no answer choices provided. Instead, you must solve the problem and fill in your answer on a special grid. Ten questions on the test will be of this type.
On the following pages are five examples of student-produced response questions. Following each question, you will find a solution and at least one way to grid in the correct answer.
It is very important for you to understand the directions for entering answers on the grid!
You will lose valuable testing time if you read the directions for the first time when you take the test. The directions are fairly simple, and the gridding technique is similar to the way other machine-readable information is entered on forms.
A primary advantage of this format is that it allows you to enter the form of the answer that you obtain, whether whole number, decimal, or fraction. For example, if you obtain 2/5, you can grid 2/5. If you obtain .4, you can grid .4. Generally, you should grid the form of the answer that you obtain naturally in solving the problem. The grid will only hold numbers that range from 0 to 9999. Decimals and fractions can also be gridded.
When there is a range of possible correct answers, your gridded response must lie within the range. For example, consider a problem for which all numbers between 4 and 5, exclusive, are correct answers. For this question, although 4.0002 is within the range (4 < x < 5), its rounded value 4.00 is not within the range and therefore would not be considered a correct answer to the problem.
Approaches to Student-Produced Responses
Decide in which column you want to begin gridding your answers before the test starts. This strategy saves time. We recommend that you grid the first (left-hand) column of the grid or that you right-justify your answers.
If the answer is zero, grid it in column 2, 3, or 4. Zero has been omitted from column 1 to encourage you to grid the most accurate values for rounded answers. For example, an answer of 1/8 could also be gridded as .125 but not as 0.12, which is less accurate.
A fraction does not have to be reduced unless it will not fit the grid. For example, 15/25 will not fit. You can grid 3/5, 6/10, or 9/15. The decimal form, .6, can also be gridded.
Do your best to be certain of your answer before you grid it. If you erase your answer, do so completely. Incomplete erasures may be picked up by the scoring machines as intended answers.
Check your work if your answer does not fit on the grid. If you obtain a negative value, a value greater than 9999, or an irrational number, you have made an error.
Make an educated guess if you don't know the answer. On student-produced response (grid-in) questions you don't lose points for wrong answers.
Always enter your answer on the grid. Only answers entered on the grid are scored. Your handwritten answer at the top of the grid isn't scored. However, writing your answer at the top of the grid may help you avoid gridding errors.
1.

What value of x satisfies both of the equations above?
2. For all positive integers a and b, let a · b be defined by

What is the value of 4 · 2 ?
3. Of the 6 courses offered by the music department at her college, Kay must choose exactly 2 of them. How many different combinations of 2 courses are possible for Kay if there are no restrictions on which 2 courses she can choose?
4. Let the function f be defined by f(x) = x2 - 7x + 10 and f(t + 1) = 0, what is one possible value of t?
5. Three parallel lines in a plane are intersected by a fourth line, forming twelve angles. If one of the angles has measure 28°, how many of the other eleven angles have measure 28°?
Answers
1.
Correct Answer:


Explanation:
Since  the value of
the value of  is either 5 or -5.
is either 5 or -5.
 or
or 
The two values of x that satisfy the first equation are 3 and 
Since  the value of 3 - 8x is either 1 or -1.
the value of 3 - 8x is either 1 or -1.
 or
or 
The two values of x that satisfy the second equation are  and
and 
You are asked to find the value of x that satisfies both equations. That value is  The answer can be entered in the grid as 1/2 or .5.
The answer can be entered in the grid as 1/2 or .5.
2.
Correct Answer:



Explanation:
The words "let a · b be defined by" tell you that the symbol · is not supposed to represent a normal mathematical operation but one that is made up for this question. To evaluate 4 · 2 you would substitute 4 for a and 2 for b in the expression  This gives
This gives  which equals
which equals  The answer may be entered in the grid as 17/3 or as 5.66 or 5.67.
The answer may be entered in the grid as 17/3 or as 5.66 or 5.67.
Correct Answer:

Explanation:
There are 6 courses offered; let us refer to them as 1, 2, 3, 4, 5, and 6. One way to find the number of combinations is to list all possible pairings. They are 1-2, 1-3, 1-4, 1-5, 1-6, 2-3, 2-4, 2-5, 2-6, 3-4, 3-5, 3-6, 4-5, 4-6, and 5-6. There are 15 combinations. Note that 1-2 and 2-1 represent the same combination.
You could also notice that there are 5 pairings that start with course 1 and 4 additional pairings that start with course 2, and so forth. The total number of combinations is 5 + 4 + 3 + 2 + 1 = 15.
You could also solve the problem by noting that the total number of permutations (that is, the number of different ways 2 of 6 courses could be selected) is 6 for the first course selected times 5 for the second course selected, or 6 × 5 = 30. To find the number of combinations, you must divide the number of permutations by the number of arrangements. For each pair of courses A-B selected, the arrangement B-A is also possible. Therefore, there are 2 arrangements. So, the number of combinations
is 30 ÷ 2 = 15.
Correct Answer:


Explanation:
Since f(x) = x2 - 7x + 10 substituting (t + 1) for x into the function yields f(t + 1) = (t + 1)2 - 7(t + 1) + 10, or f(t + 1) = (t2 + 2t + 1) - (7t + 7) + 10, or f(t + 1) = t2 - 5t + 4. Therefore t = 1 or t = 4.
Since f(t + 1) = 0, it follows that t2 - 5t + 4 = 0, or (t - 1)(t - 4) = 0. Therefore t = 1 or t = 4.
Another way to solve the question would be to use a dummy variable k. For example, let k = t + 1. f(k) = k2 - 7k + 10 = (k - 5)(k - 2). Since k = t + 1 it follows that f(k) = 0. So (k - 5)(k - 2) = 0, and therefore, k= 5 or k = 2. Since t = k - 1, t = 4 or t = 1.
This question asks for one possible value of x. Either 1 or 4 (not both) satisfy the question being asked. Choose only one correct answer to enter in the grid.
When there is a range of possible correct answers, your gridded response must lie within the range. For example, consider a problem for which all numbers between 4 and 5, exclusive, are correct answers. For this problem, although 4.0002 is within the range (4 < t < 5), its rounded value 4.00 is not within the range and therefore would not be considered a correct answer to the problem.
5.
Correct Answer:

Explanation:
Drawing the figure described in the problem will help you visualize the correct solution to the problem. The figure below shows three parallel lines intersected by a fourth line. The acute angle is labeled 28°, as shown below.

Using the fact that vertical angles and alternate interior angles are equal, you can put a check showing the other angles in the figure that also measure 28°, as shown below.

There are 5 other angles that measure 28°. Therefore, the correct answer to this problem is 5. The number 5 can be gridded in any of the four columns on the answer grid.
'SAT II Math (Subject Test)' 카테고리의 다른 글
| SAT Math Level2 book - 시험대비용 (0) | 2009.04.24 |
|---|---|
| 2008-09 The SAT® Subject Test Preparation Booklet (0) | 2009.03.22 |
| Canada Grade 12 Math MB (0) | 2009.02.06 |
| Canada Grade 12 Math AB (0) | 2009.02.06 |